Abstract
(Talk in Spanish)
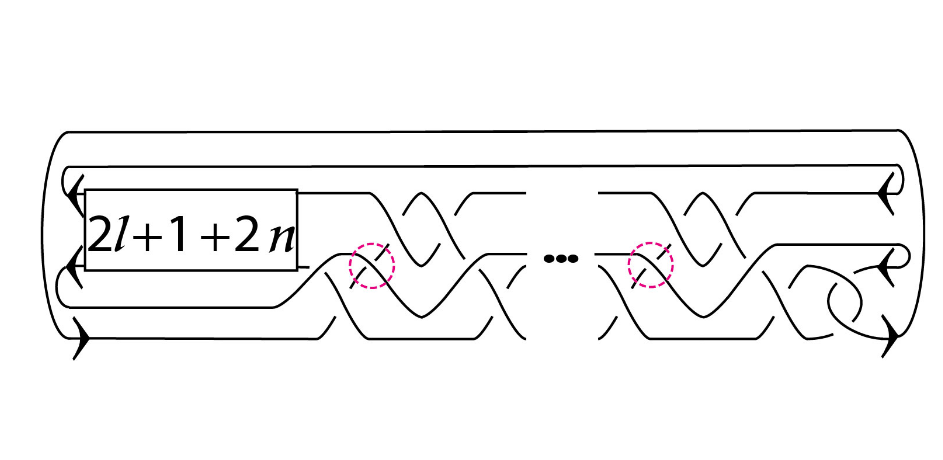
Due to Alexander’s theorem, it is well known that any link can be represented as a closed braid. Besides, a link is alternating or non-alternating depending on whether it possesses an alternating diagram or not. However, there are alternating links that cannot be represented as alternating closed braids. In this talk, we shall discuss the set of links that can be represented as alternating closed braids, and we shall introduce invariants that measure how far the links are from this set. We will show the relations of these invariants with others as the unknotting number and the alternation number. Furthermore, we will give the value of these invariants for some knot families. This work is partially joint with A. Kawauchi.